Question

Which of the graphs above is the graph of the equation below?

y = 13 – 632 + 111 - 6 = (1 - 3)(1 – 2)(1 - 1)

Answer 1

w

Explanation:

for Plato

Answer 2

Shown below

Explanation:

To solve this problem, we need to analyze the leading coefficient and the roots of the polynomial function:

`f(x)=x^3-6x^2+11x-6`

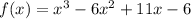
Recall that a polynomial function can be represented by:

`f(x)=a_(n)(x)+ \ldots +a_(1)x+a_(0)`

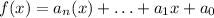
So the leading coefficient is
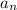`a_(n)`. In our problem, this coefficient is
`a_(n)=1`

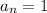
Since
`n` is odd and the leading coefficient
`a_(n)>0`, then the graph must falls to the left and rise to the right. Also, the roots are
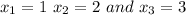`x_(1)=1 \ x_(2)=2 \ and \ x_(3)=3`. So the only graph that matches this is the fourth one as indicated below.