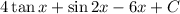Question

Evaluate the integral following ​

Answer 1

`\displaystyle{4\tan x + \sin 2x - 6x + C}`

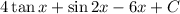
Explanation:

We are given the integral of:

`\displaystyle{\int 4(\sec x - \cos x)^2 \, dx}`

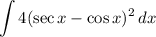
First, we can use a property to separate a constant out of integrand:

`\displaystyle{4 \int (\sec x - \cos x)^2 \, dx}`

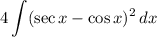
Next, expand the expression (integrand):

`\displaystyle{4 \int \sec^2 x - 2\sec x \cos x + \cos^2 x \, dx}`

Since
`\displaystyle{\sec x = (1)/(\cos x)}` then it can be simplified to:

`\displaystyle{4 \int (1)/(\cos^2 x) - 2(1)/(\cos x) \cos x + \cos^2 x \, dx}\\\\\displaystyle{4 \int (1)/(\cos^2 x) - 2 + \cos^2 x \, dx}`

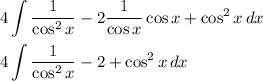
Recall the formula:

`\displaystyle{\int (1)/(\cos ^2 x) \, dx = \int \sec ^2 x \, dx = \tan x + C}\\\\\displaystyle{\int A \, dx = Ax + C \ \ \tt{(A \ and \ C \ are \ constant.)}`

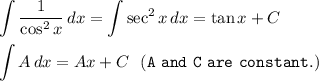
For
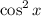`\displaystyle{\cos ^2 x}`, we need to convert to another identity since the integrand does not have a default or specific integration formula. We know that:

`\displaystyle{2\cos^2 x -1 = \cos2x}`

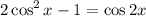
We can solve for
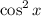`\displaystyle{\cos ^2x}` which is:

`\displaystyle{2\cos^2 x = \cos2x+1}\\\\\displaystyle{\cos^2x = (\cos 2x +1)/(2)}`

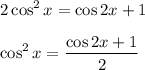
Therefore, we can write new integral as:

`\displaystyle{4 \int (1)/(\cos^2 x) - 2 + (\cos2x +1)/(2) \, dx}`

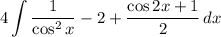
Evaluate each integral, applying the integration formula:

`\displaystyle{\int (1)/(\cos^2x) \, dx = \boxed{\tan x + C}}\\\\\displaystyle{\int -2 \, dx = \boxed{-2x + C}}\\\\\displaystyle{\int (\cos 2x +1)/(2) \, dx = (1)/(2)\int \cos 2x +1 \, dx}\\\\\displaystyle{= (1)/(2)\left((1)/(2)\sin 2x + x\right) + C}\\\\\displaystyle{= \boxed{(1)/(4)\sin 2x + (1)/(2)x + C}}`

Then add all these boxed integrated together then we'll get:

`\displaystyle{4\left(\tan x - 2x + (1)/(4)\sin 2x + (1)/(2) x\right) + C}`

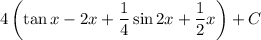
Expand 4 in the expression:

`\displaystyle{4\tan x - 8x +\sin 2x + 2 x + C}\\\\\displaystyle{4\tan x + \sin 2x - 6x + C}`

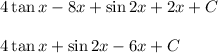
Therefore, the answer is:

`\displaystyle{4\tan x + \sin 2x - 6x + C}`