Hello!
The answer is:
The sum the other angles that do not measure 22° is equal to 316°.
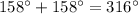
Why?
To find the sum of the rest of the angles, we need to remember that a rhombus has two adjacent and equal angles, and also it has two opposite and equal angles.
So, we are given that the rhombus has an angle of 22°, and to calculate the other angles, let be the given angle an adjacent angle, so:

Hence, we have that the sum of its other angles that do not measure 22° is equal to 316°.
Have a nice day!