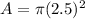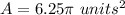Question

The endpoints of a diameter of a circle are A(2,1) and B(5,5). Find the area of the circle in terms of pi.

Answer 1

The area of the circle is
`A=6.25\pi\ units^(2)`

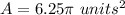
Explanation:

step 1

Find the diameter of circle

we know that

The diameter of the circle is equal to the distance AB

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

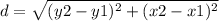
substitute the values

`AB=\sqrt{(5-1)^(2)+(5-2)^(2)}`

`AB=\sqrt{(4)^(2)+(3)^(2)}`

`AB=√(25)`

`AB=5\ units`

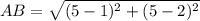
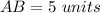
therefore

the diameter of the circle is

`D=5\ units`

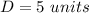
step 2

Find the area of the circle

The area of the circle is equal to

`A=\pi r^(2)`

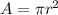
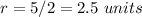`r=5/2=2.5\ units` ----> the radius is half the diameter

substitute

`A=\pi (2.5)^(2)`

`A=6.25\pi\ units^(2)`