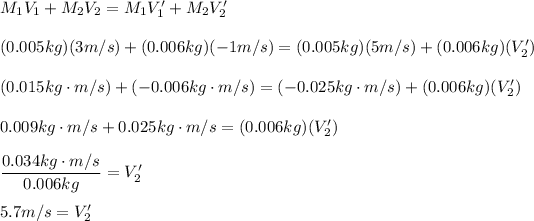Question

Two billiard balls move toward each other on a table. The mass of the number three ball, m1, is 5 g with a velocity of 3 m/s. The mass of the eight ball, m2, is 6 g with a velocity of 1 m/s. After the balls collide, they bounce off each other. The number three ball moves off with a velocity of 5 m/s. What is the final velocity and direction of the eight ball? +8.6 m/s +5.7 m/s –5.7 m/s –8.6 m/s.?

Answer 1

+5.7 m/s

Step-by-step explanation:

Just did the assignment :)

Answer 2

+5.7 m/s

Step-by-step explanation:

According to the law of conservation of momentum is that the momentum before the collision is equal to the momentum after the collision. In an equation form it would look like this:

M₁V₁+M₂V₂ = M₁V₁'+M₂V₂'

Where:

M₁ = mass of object 1 (kg)

V₁ = velocity of object 1 before the collision (m/s)

V₁' = Final velocity of object 1 after the collision (m/s)

M₂ = mass of object 2 (kg)

V₂ = velocity of object 2 before the collision (m/s)

V₂' = Final velocity of object 2 after the collision (m/s)

According to your problem you have the following given:

M₁ = 5 g = 0.005kg

V₁ = 3 m/s

V₁' = -5m/s (It bounced off so it is going the other direction)

M₂ = 6g = 0.006kg

V₂ = -1 m/s (It is coming from the opposite direction of the 3-ball)

V₂' = ?

So we plug in what we know and solve for what we don't know.

`M_1V_1+M_2V_2 = M_1V_1' + M_2V_2'\\\\(0.005kg)(3m/s)+(0.006kg)(-1m/s) = (0.005kg)(5m/s)+(0.006kg)(V_2')\\\\(0.015kg\cdot m/s)+(-0.006kg\cdot m/s)=(-0.025kg\cdot m/s)+(0.006kg)(V_2')\\\\0.009kg\cdot m/s+0.025kg\cdot m/s = (0.006kg)(V_2')\\\\(0.034kg\cdot m/s)/(0.006kg) = V_2'\\\\5.7m/s = V_2'`