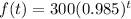Answer:
Part 1) The simple interest earned when you invest $1,000 for 3 years at 10 % is $300
Part 2) The interest compounded when you invest the same sum for 2 years at 5 % is $102.50
Part 3)

Part 4)
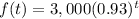
Part 5)
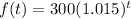
Part 6)
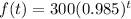
Explanation:
Part 1) What will be the simple interest earned when you invest $1,000 for 3 years at 10 percent
we know that
The simple interest formula is equal to
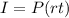
where
I is the Final Interest Value
P is the Principal amount of money to be invested
r is the rate of interest
t is Number of Time Periods
in this problem we have
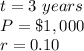
substitute in the formula above
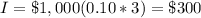
Part 2) What will be the compound interest earned when you invest $1,000 for 2 years at 5 percent ?
we know that
The compound interest formula is equal to

where
I is the Final Investment Value
P is the Principal amount of money to be invested
r is the rate of interest in decimal
t is Number of Time Periods
n is the number of times interest is compounded per year
in this problem we have
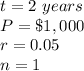
substitute in the formula above


The interest is equal to
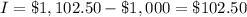
Part 3) There are 7 trout fish in a pond, and the population doubles every year.
Find the population after t years.
we know that
This question is about exponent function of the form
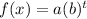
where
a is the initial value
b is the base of the exponent.
In this problem we have
There are 7 trout fish in the pound ----> initial value a=7
The population is double every year ------> the base is b=2
substitute
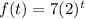
Part 4) A company buys a machine for $3,000. The value of the machine depreciates by 7% every year. Find the value of the machine after t years.
we know that
This question is about exponent function of the form
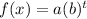
where
a is the initial value
b is the base of the exponent.
we have
Company buys a machine for $3,000 --> initial value is a=3,000
The value depreciate 7% a year
Since it was decreased by 7% every year, it will become: 100%-7%=93%
the base is 93%, b=0.93
substitute
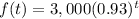
Part 5) The initial population of a colony of ants is 300. The number of ants increases at a rate of 1.5% every month. Find the population of ants after t months.
we know that
This question is about exponent function of the form
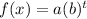
where
a is the initial value
b is the base of the exponent.
we have
Initial population of ants is 300----> initial value is a=300
The number of ants increases 1.5% per month.
Since it will increases by 1.5% every month, it will become: 100%+1.5%=101.5%
the base is 101.5%, b=1.015
substitute
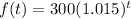
Part 6) A research laboratory is testing a new vaccine on 300 infected cells. The decay rate is 1.5% per minute. Find the number of infected cells after t minutes.
we know that
This question is about exponent function of the form
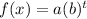
where
a is the initial value
b is the base of the exponent.
we have
A research laboratory is testing new vaccine on 300 infected cells
initial value is a=300
The decay/decrease rate is 1.5% per minute
Since it will decrease by 1.5% every min, it will become: 100%-1.5%=98.5%
the base is 98.5%, b=0.985
substitute