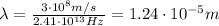Answer:
Frequency:
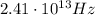 , Wavelength:
, Wavelength:
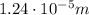
Step-by-step explanation:
The energy of the photon is equal to the energy required to break the bond, so 0.1 eV.
First of all, we need to convert the energy of the photon from eV to Joule:
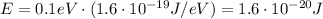
The energy of the photon is related to its frequency by:
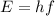
where h is the Planck constant and f is the frequency.
Solving for f,
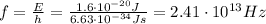
The wavelength instead is given by
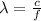
where c is the speed of light. Substituting,