Question

Factor to the given polynomial completely.

4x³+24x²-288x

Answer 1

4x(x + 6)(x - 12).

Explanation:

First take out 4x which is the GCF of the 3 terms:

= 4x (x^2 - 6x - 72)

We need 2 numbers whose product is -72 and whose sum is -6.

They are -12 and + 6 so we have:

4x(x + 6)(x - 12)

Answer 2

Answer:
`\Large\boxed{4x(x+12)(x-6)}`

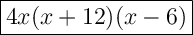
Explanation:

Given expression

4x³ + 24x² - 288x

Factorize 4x out from the expression

4x · x² + 4x · 6x - 4x · 72

4x (x² + 6x - 72)

Cross multiply to factorize the remaining polynomial expression

The meaning is to allow the factored product of the constant to add up to the first-degree term

x 12

x -6

Combine the result

`\Large\boxed{4x(x+12)(x-6)}`

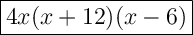
Hope this helps!! :)

Please let me know if you have any questions