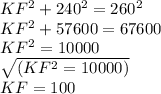Question

Erin is flying a kite. She knows that 260 feet of the kite

string has been let out. Also, a friend is 240 feet away
and standing directly underneath the kite. How high off
the ground is the kite?

Answer 1

100 ft high

Explanation:

We know that Erin, the kite, and her friend create a right triangle since the friend is standing directly under the kite and in front of Erin.

Thus, we can use the Pythagorean theorem to find the height of the kite.

We can let the side the distance be at represents Erin and the kite's distance be EK (aka the hypotenuse), the side that represents Erin and her friend's distance be EF and the side that represents the kite and the friend's distance be KF.

The theorem says that a^2 + b^2 = c^2, where a and b are the two shortest sides and c is the hypotenuse.

We already have the measure of the hypotenuse (260 ft) and one of the shorter sides (240), so we have:

`KF^2+240^2=260^2\\KF^2+57600=67600\\KF^2=10000\\√((KF^2=10000)) \\KF=100`