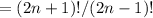Answer:
4n²+2n as a factorial is given as:
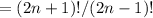
Explanation:
We are given an expression which has to be converted into factorial form.
The expression is as follows:
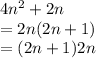
Now we know that 2n+1 and 2n differs by '1' and the next smaller term is '2n-1'.
Hence, multiplying and dividing by '(2n-1)!'; we get:
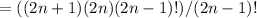
we know that x(x-1)(x-2)! = x!, so: