Question

Find all polar coordinates of point P where P = ordered pair 6 comma negative pi divided by 5.

Answer 1

P(6,-π/6) = (6, -π/6 + 2nπ) and P(-6,-π/6) = (-6, -π/6 + (2n+1)π)

Explanation:

We need to find all polar coordinates of

`P = (6,(-\pi )/(6))`

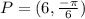
The polar coordinates of any point can be reresented by (r,Ф)

where

(r,Ф) = (r, Ф+2nπ) where n is any integer and r is positive

and

(r,Ф) = (-r, Ф+(2n+1)π) where n is any integer and r is negative.

So, in the question given, r = 6 and Ф = -π/6

So, Polar coordinates will be:

P(6,-π/6) = (6, -π/6 + 2nπ) where where n is any integer and r is positive

and

P(-6,-π/6) = (-6, -π/6 + (2n+1)π) where n is any integer and r is negative.

Answer 2

All polar coordinates of point P are
`(6,-(\pi)/(5)+2n\pi)` and
`(-6,-(\pi)/(5)+(2n+1)\pi)`, where n is an integer.

Explanation:

The given point is

`P=(6,-(\pi)/(5))`

If a point is
`P=(r,\theta)`, then all polar coordinates of point P are defined as

`(r,\theta)=(r,\theta+2n\pi)`

`(r,\theta)=(-r,\theta-(2n+1)\pi)`

where n is an integer.

In the given point
`r=6` and
`\theta=-(\pi)/(5)`. So all polar coordinates of point P are defined as

`(6,-(\pi)/(5))=(6,-(\pi)/(5)+2n\pi)`

`(6,-(\pi)/(5))=(-6,-(\pi)/(5)+(2n+1)\pi)`

Therefore all polar coordinates of point P are
`(6,-(\pi)/(5)+2n\pi)` and
`(-6,-(\pi)/(5)+(2n+1)\pi)`, where n is an integer.