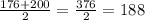Question

Find the values of the 30th and 90th percentiles of the data. Please show your work.

129, 113, 200, 100, 105, 132, 100, 176, 146, 152

Answer 1

30th percentile:109

90th percentile: 188

Explanation:

The given data set is:

129, 113, 200, 100, 105, 132, 100, 176, 146, 152

We arrange the data set in ascending order to obtain;

Array=
`100,100,105,113,129,132,146,176,200`

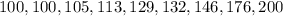
The 30th percentile is located at

`((30)/(100)* 10 )}` -th position, where n=10 is the number of items in the data set

This implies that the 30th percentile is at the 3rd position.

Since 3 is an integer, the 30th percentile is the average of the 3rd and 4th occurrence in the array;

This implies that the 30th percentile =
`(105+113)/(2)=(218)/(2)=109`

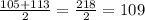
The 90th percentile is located at

`((90)/(100)* 10 )}` -th position, where n=10 is the number of items in the data set

This implies that the 90th percentile is at the 9th position.

Since 9 is an integer, the 90th percentile is the average of the 9th and 10th occurrence in the array;

This implies that the 90th percentile =
`(176+200)/(2)=(376)/(2)=188`