Answer:
B. 99 square units
Explanation:
The parallelogram is a quadrilateral where the opposite sides are parallel to each other. This geometric figure constitutes, therefore, a polygon that consists of 4 sides where there are two cases of parallel sides.
To calculate the area of a parallelogram, you must know the length of the height relative to one of its sides.
Let the base be the side x and the height (h) relative to the base. The area of the parallelogram is the product of the base and the height.
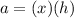 Where:
Where:
a is the area in square units.
x is the base in units.
h is the height in units.
Solving:
With x = 11 units and h = 9 units
