Question

1.50 moles of N2 at 825 mmhg and 303 K are contained in a 34.3 L bottle. What is the pressure of the system if an additional 1.00 mole of gas is added to the bottle and the temperature is reduced to 273 K?

Answer 1

Answer:1240

Step-by-step explanation:

Answer 2

1240 mmHg

Step-by-step explanation:

Since volume is being held constant, we can use the following variation of the Ideal Gas Law to find the new pressure.

`(P_1)/(T_1N_1)=(P_2)/(T_2N_2)`

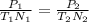
In the equation, "P₁", "T₁", and "N₁" represent the initial pressure, temperature, and moles. "P₂", "T₂", and "N₂" represent the final pressure, temperature, and moles. Your answer should have 3 sig figs to match the sig figs of the given values.

P₁ = 825 mmHg P₂ = ? mmHg

T₁ = 303 K T₂ = 273 K

N₁ = 1.50 moles N₂ = 1.50 + 1.00 = 2.50 moles

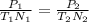`(P_1)/(T_1N_1)=(P_2)/(T_2N_2)` <----- Formula

`(825 mmHg)/((303K)(1.50 moles))=(P_2)/((273 K)(2.50 moles))` <----- Insert values

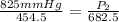`(825 mmHg)/(454.5)=(P_2)/(682.5)` <----- Simplify denominators

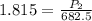`1.815=(P_2)/(682.5)` <----- Simplify left side

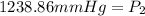`1238.86 mmHg={P_2}` <----- Multiply both sides by 682.5

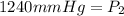`1240 mmHg={P_2}` <----- Apply sig figs