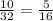Answer:
The probability is
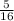
Explanation:
Given is that a fair coin is tossed 5 times in a row.
This gives total number of outcomes as =
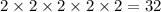
As its needed that heads comes exactly 2 times, so favorable outcomes are = 5C2
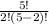
Solving this we get 10
Therefore, the probability of getting heads exactly 2 times is =