Answer: Option B
Explanation:
By definition, the ratio is a comparison between two different things. It can be written in:
Odd notation

Fractional notation
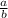
Or in words:
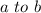
Given the ratio 3 to 4 and the ratio of 4 to 3, you can rewrite them the fractional form:

To know if these ratios are the same number, divide the numerator by the denominator of each one of them:
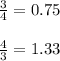
Therefore, the ratio of 3 to 4 and the ratio of 4 to 3 ARE NOT the same number.