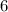Question

A discount bookstore sells hardcover books for $12 and paperback books for $6. The number of hardcover books, x, sold one day was twice the number of paperback books, y, sold the same day. The total sales that day were $180.

1.What is the system of equations that represents this situation?


2.What is the solution to the system and what does it represent in the context of this situation? Show your work.

Answer 1

Part 1) The system of equations that represent this situation is

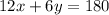`12x+6y=180` and
`x=2y`

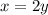
Part 2) The number of hardcover books sold was
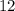`12` and the number of paperback books sold was
`6`

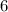
Explanation:

Part 1) What is the system of equations that represents this situation?

Let

x----> number of hardcover books sold

y----> number of paperback books sold

we know that

The system of equations that represent this situation is equal to

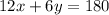`12x+6y=180` -----> equation A

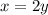`x=2y` -----> equation B

Part 2) What is the solution to the system and what does it represent in the context of this situation?

Substitute equation B in equation A and solve for y

`12(2y)+6y=180`

`24y+6y=180`

`30y=180`

`y=6`

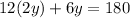
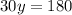
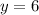
Find the value of x

`x=2y`

`x=2(6)=12`

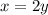
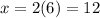
The solution of the system of equations is the point (12,6)

That means

The number of hardcover books sold was
`12`

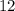
The number of paperback books sold was
`6`