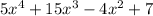Question

Simplify each expression


`(7x ^(3) + 5x ^(4) + 3x ^(2) ) - (7 {x}^(2) - 7 - 8 {x}^(3) )`
​

Answer 1

`5x^4 + 15x^3 - 4x^2 + 7`

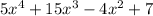
Explanation:

`(7x^3 + 5x^4 + 3x^2) - (7x^2 - 7 -8x^3)`

what we need to do is distribute the - sign in the middle of the parentheses into (7x^2 - 7 -8x^3)[/tex], this is very important to ensure we have the correct answer. by distributing we get the following equation:

`7x^3 + 5x^4 + 3x^2 - 7x^2 + 7 + 8x^3`

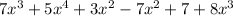
now we combine like terms to get our answer

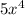`5x^4` stays as it is, since there is nothing to combine it with

7x³ + 8x³ = 15x³

3x² - 7x² = -4x²

7 stays the same because like
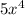`5x^4`, there is nothing else to combine it with

we have the following new equation:

`5x^4 + 15x^3 - 4x^2 + 7`