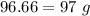Question

Helppleaseee!!! It's due tomorrow??!!​

Answer 1

Part a) The total length of the circular portions is
`12\pi\ in`

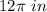
Part b) The total length of wire needed is tex]53.7\ in[/tex]

Part c) The total weight of the ornament is
`97\ g`

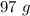
Explanation:

Part a) Find the total length of the circular portions in terms of pi

we know that

The diameter of the circle is equal to the length side of the square

`D=4\ in`

The total length of the circular portions is equal to the circumference of three complete circle

Because each circular portion is equal to 3/4 of circle

so

`(3/4)*4=3`

The circumference of four three quarter circles is equal to

`C=3(\pi D)`

substitute the diameter

`C=3(\pi (4))`

`C=12\pi\ in`

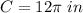
Part b) Find the total length of wire needed

The total length of the circular portions is
`12\pi\ in`

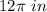
assume

`\pi =3.14`

`12(3.14)=37.68\ in`

The total length of the square portion is equal to

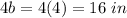`4b=4(4)=16\ in` -----> perimeter of a square

Adds the lengths

`37.68+16=53.68\ in`

Round to the nearest tenth

`53.68=53.7\ in`

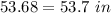
Part c) Find the total weight of the ornament

we know that the ornament weights 1.8 grams per inch

so

Multiply the total length by 1.8 to obtain the total weight

`53.7(1.8)=96.66\ g`

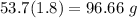
Round to the nearest gram

`96.66=97\ g`