Question

Waves with an amplitude of 2ft pass a doc every 30 seconds. Write an equation for a cosine model the height of a water particle above and below the mean water line

Answer 1

`\bf ~~~~~~~~~~~~\textit{function transformations} \\\\\\ f(x)=Asin(Bx+C)+D \qquad \qquad f(x)=Acos(Bx+C)+D \\\\ f(x)=Atan(Bx+C)+D \qquad \qquad f(x)=Asec(Bx+C)+D \\\\[-0.35em] ~\dotfill\\\\ \bullet \textit{ stretches or shrinks}\\ ~~~~~~\textit{horizontally by amplitude } A\cdot B\\\\ \bullet \textit{ flips it upside-down if }A\textit{ is negative}`

`\bf ~~~~~~\textit{reflection over the x-axis} \\\\ \bullet \textit{ flips it sideways if }B\textit{ is negative}\\ ~~~~~~\textit{reflection over the y-axis} \\\\ \bullet \textit{ horizontal shift by }(C)/(B)\\ ~~~~~~if\ (C)/(B)\textit{ is negative, to the right}\\\\ ~~~~~~if\ (C)/(B)\textit{ is positive, to the left}`

`\bf \bullet \textit{vertical shift by }D\\ ~~~~~~if\ D\textit{ is negative, downwards}\\\\ ~~~~~~if\ D\textit{ is positive, upwards}\\\\ \bullet \textit{function period or frequency}\\ ~~~~~~(2\pi )/(B)\ for\ cos(\theta),\ sin(\theta),\ sec(\theta),\ csc(\theta)\\\\ ~~~~~~(\pi )/(B)\ for\ tan(\theta),\ cot(\theta)`

with that template in mind, let's see.

`\bf \stackrel{A = 2}{g(x) = 2cos(Bx+C)}\qquad \begin{cases} \stackrel{\textit{period of 30 seconds}}{\cfrac{2\pi }{B}=30}\\\\ \cfrac{2\pi }{30}=B\\\\ \cfrac{\pi }{15}=B \end{cases}\implies g(x)=2cos\left( (\pi )/(15)x+C \right)`

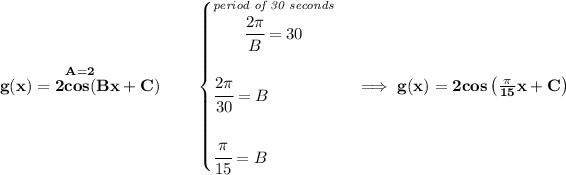
now, since the period is 30, and cos(x) starts off at 1, recall cos(0) = 1, so then le'ts move the starting point over by simply doing a horizontal shift to the right by a quarter of the period, 30/4 = 15/2 units, that way the initial "hump" starts off at 0.

`\bf \stackrel{\textit{horizontal shift of }(15)/(2)}{\cfrac{15}{2}=\cfrac{C}{B}}\implies \cfrac{15}{2}=\cfrac{C}{~~(\pi )/(15)~~}\implies \cfrac{15}{2}=\cfrac{15C}{\pi }\implies 15\pi =30C \\\\\\ \cfrac{15\pi }{30}=C\implies \cfrac{\pi }{2}=C~\hfill \stackrel{\textit{horizontally to the right}}{-\cfrac{\pi }{2}=C} \\\\[-0.35em] \rule{34em}{0.25pt}\\\\ ~\hfill g(x)=2cos\left((\pi )/(15)x-(\pi )/(2) \right)~\hfill`

Check the picture below.