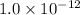Question

For the reaction cu2s(s) ⇌ 2cu+(aq) + s2- (aq), the equilibrium concentrations are as follows: [cu+ ] = 1.0 × 10-5 m, [s2-] = 1.0 × 10-2 m. the equilibrium constant is:

Answer 1

1.0 x 10⁻¹².

Step-by-step explanation:

• For the reaction:

Cu₂S(s) ⇌ 2Cu⁺(aq) + S²⁻(aq),

The equilibrium constant (Keq) = [Cu⁺]²[S²⁻]/[Cu₂S].

[Cu⁺] = 1.0 × 10⁻⁵ M, [S²⁻] = 1.0 × 10⁻² M.

[Cu₂S] = 1.0, since the concentration of solid is always can be considered = 1.0.

∴ Keq = [Cu⁺][S²⁻]/[Cu₂S] = (1.0 × 10⁻⁵)²(1.0 × 10⁻²)/(1.0) = 1.0 x 10⁻¹².

Answer 2

Answer: The equilibrium constant for the above reaction is
`1.0* 10^(-12)`

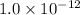
Step-by-step explanation:

Equilibrium constant in terms of concentration is defined as the ratio of concentration of products to the concentration of reactants each raised to the power their stoichiometric ratios. It is expressed as
`K_(c)`

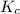
For a general chemical reaction:

`aA+bB\rightarrow cC+dD`

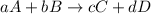
The expression for
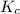`K_(c)` is written as:

`K_(c)=([C]^c[D]^d)/([A]^a[B]^b)`

Concentration of solid and liquid substances in a chemical reaction is taken to be 1.

For the given chemical reaction:

`Cu_2S(s)\rightarrow 2Cu^+(aq.)+S^(2-)(aq.)`

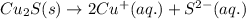
The expression for
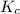`K_(c)` is written follows:

`K_c=[Cu^+]^2* [S^(2-)]`

We are given:

`[Cu^+]=1.0* 10^(-5)M`

`[S^(2-)]=1.0* 10^(-2)M`

Putting values in above expression, we get:

`K_c=(1.0* 10^(-5))^2* (1.0* 10^(-2))\\\\K_c=1.0* 10^(-12)`

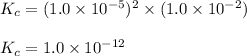
Hence, the equilibrium constant for the above reaction is
`1.0* 10^(-12)`