Question

Which type of sequence is represented by the given table?

x
1
2
3
4
y
4
-9.6
23.04
-55.296

A.
The table represents a geometric sequence because the successive y-values have a common ratio of -2.4.
B.
The table represents an arithmetic sequence because the successive y-values have a common difference of -17.
C.
The table represents a geometric sequence because the successive y-values have a common ratio of 0.4.
D.
The table represents an arithmetic sequence because the successive y-values have a common difference of 4.2.

Answer 1

The table represents a geometric sequence because the successive y-values have a common ratio of -2.4

Answer 2

A.

The table represents a geometric sequence because the successive y-values have a common ratio of -2.4.

Explanation:

A geometric sequence, with a first term a and common ratio r, is generally represented as;

`a,ar,ar^(2),ar^(3),ar^(4),............ar^(n)`

The first term refers to the first number that appears in the sequence. The common ratio is the constant that multiplies a preceding value to obtain the successive one. That is, to obtain
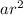`ar^(2)` from
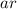`ar` we multiply
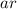`ar` by the common ratio r.

In the table given the y-values are as follows;

4, -9.6, 23.04, -55.296

To obtain the common ratio we simply divide each value by the preceding one;

(-9.6)/4 = -2.4

23.04/(-9.6) = -2.4

(-55.296)/23.04 = -2.4

Since the sequence of numbers has a common ratio then it qualifies to be a geometric sequence. Thus, the table represents a geometric sequence because the successive y-values have a common ratio of -2.4.