Question

Determine the domain of the function. f as a function of x is equal to the square root of x plus one divided by x plus four times x minus six.

answers:
a) x ≥ -1, x ≠ 6
b) All real numbers except -4 and 6
c) All real numbers
d) x ≥ 0

Answer 1

a)
`x\geq -1,x\\eq 6`

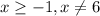
Explanation:

We have been given a function
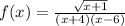`f(x)=(√(x+1))/((x+4)(x-6))`. We are asked to find the domain of our given function.

We can see that our given function is a rational function and numerator of our given function is a square root.

To find the domain of our given function, we will find the number that will make our denominator 0 and the domain of square root function will be the values of x that will make our numerator non-negative.

Undefined points for our given function:

`(x+4)(x-6)=0`

`x+4=0\text{ or }x-6=0`

`x=-4\text{ or }x=6`

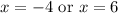
The domain of denominator is all values of x, where x is not equal to negative 4 and positive 6.

Non negative values for radical:

`x+1\geq 0`

`x+1-1\geq 0-1`

`x\geq -1`

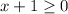
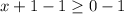
The domain of numerator is all value of x greater than or equal to negative

Upon combining real regions and undefined points for our given function, the domain of our given function will be all values of x greater than or equal to negative 1, where x is not defined for 6.

Therefore, domain of our given function is
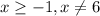`x\geq -1,x\\eq 6`.

Answer 2

a) x ≥ -1 , x ≠ 6

Explanation:

To quickly solve this problem, we can use a graphing tool or a calculator to plot the equation.

Please see the attached images below, to find more information about the graph

The correct answer is option

a) x ≥ -1 , x ≠ 6