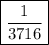Answer:
1.
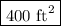 ; 2.
; 2.
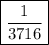
Explanation:
Step 1. Calculate the area of the floor
If the area A₁ of a square floor on the scale drawing is 100 cm², the length of a side is 10 cm.
The side length l of the actual floor is
l = 10 cm × (2 ft/1 cm) = 20 ft
The area A₂ of the floor is
A = l² = (20 ft)² =

Step 2. Calculate the area ratios
We must express both areas in the same units.
Let's express the area of the room in square centimetres.
l = 20 ft × (12 in/1 ft) = 240 in
l = 240 in × (2.54 cm/1 in) = 609.6 cm
A₂ = l² = (609.6 cm)² = 371 612 cm²
The area A₁ on the scale drawing is 100 cm².
The ratio of the areas is

The ratio of the area in the drawing to the actual area is