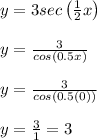Question

Choose the function whose graph is given by:

Answer 1

`y=3sec\left((1)/(2)x\right)`

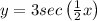
Explanation:

The graphs of
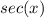`sec(x)` can be obtained from the graph of the cosine function using the reciprocal identity, so:

`sec(x)=(1)/(cos(x))`

But in this problem, the graph stands for the function:

`y=3sec\left((1)/(2)x\right)`

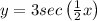
Because the period is now 4π as indicated and for
`x=0` in the figure and this can be proven as follows:

`Period=(2\pi)/((1)/(2))=4\pi`

Also,
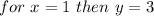`for \ x=1 \ then \ y=3` as indicated in the figure and this can be proven as:

`y=3sec\left((1)/(2)x\right) \\ \\ y=(3)/(cos(0.5x)) \\ \\ y=(3)/(cos(0.5(0))) \\ \\ y=(3)/(1)=3`