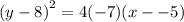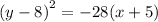Question

Please Help

Write the equation of a parabola with vertex (-5,8) and directrix x=2. Show all of your work and put your equation in graphing/vertex form.

Answer 1

`( {y - 8)}^(2) = - 28(x + 5)`

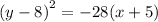
Step-by-step explanation

The given parabola has directrix x=2.

This implies that, the parabola is opens in the direction of the negative x-axis because it must open in a negative direction to the directrix.

The equation of such parabola is of the form:

`( {y - k)}^(2) = 4p(x - h)`

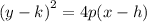
where (h,k)=(-5,8) is the vertex.

`|p| = | - 2 - 5| = 7`

`p = \pm7`

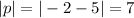
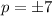
But the parabola opens to the left.

p=-7

The equation now becomes

`( {y - 8)}^(2) = 4( - 7)(x - - 5)`

`( {y - 8)}^(2) = - 28(x + 5)`