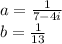Question

Type the correct answer in each box. If necessary, use / for the fraction bar.

Complete the statement.

​

Answer 1

a= 7b/5 + 4bi/5

b= -4ai/13 + 7a/13

Explanation:

Answer 2

The answers are:

`a=(1)/(7-4i) \\b=(1)/(13)`

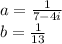
Explanation:

In order to determine the values of both a and b variables, we have to know a property of the imaginary numbers. The imaginary unit,
`i` , has the following rule:

`i=√(-1)\\i^2=i*i=-1`

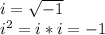
Then, we add both fraction inside of the parenthesis:

`(3+2i)/(2-3i) +(5-i)/(2+3i)\\((3+2i)*(2+3i)+(5-i)*(2-3i))/((2-3i)*(2+3i))\\(6+9i+4i+6i^2+10-15i-2i+3i^2)/(4+6i-6i-9i^2)\\(16-4i+9i^2)/(4-9i^2)\\(16-4i+9*(-1))/(4-9*(-1))\\(7-4i)/(13)`

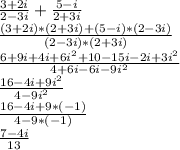
Then, if we multiply the numerator by a, we have to get 1. Then if we multiply by b the denominator, we have to get 1. So:

`a=(1)/(7-4i) \\b=(1)/(13)`