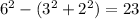Answer:
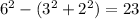
Explanation:
We have got 3 squares here. The shaded area is not clear but it is an understood fact that the question must be asking about the area in the bigger square minus the sum of smaller 2 squares.
So, the area of the bigger square is =
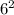 (exponent form)
(exponent form)
or 36 square inches
Area of smaller square with side 3 inches =
 (exponent form)
(exponent form)
or 9 square inches
Area of second smaller square with side 2 inches =
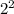 (exponent form) or 4 square inches.
(exponent form) or 4 square inches.
Sum of the area of smaller squares =
 = 9+4 = 13 sq inches
= 9+4 = 13 sq inches
And area of the shaded region will be = 36-13 = 23 square inches.
In exponent form it can be written as :