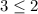Answer:
The correct answer option is C. (4, 4).
Explanation:
We are given the following equation and we are to determine whether which of the given point satisfies it:
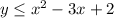
So checking by substituting the points.
A. (2, 2):
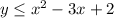 --->
--->
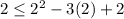 --->
--->
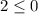
B. (1, 1):
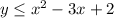 --->
--->
 --->
--->
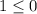
C. (4, 4):
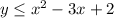 --->
--->
 --->
--->
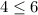 - True
- True
D. (3, 3):
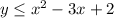 --->
--->
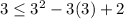 --->
--->