Answer:

Explanation:
For the function
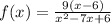 , you need to factor the denominator.
, you need to factor the denominator.
Find two number that when you add them you get -7 and when you multiply them you get 6. These numbers are -6 and -1.
Then:
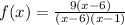
Simplify:
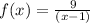
Observe that the denominator is equal to zero in
 .
.
Therefore, when x approaches 1, f(x) tends to infinite.
Then the vertical asymptote s:
