Question

Lindor's Truffles have an outer shell diameter of 1.25 in. and a creamy milk chocolate fudge that makes up the inner circle of the ball that has a diameter of 0.5 in. How much more volume does the outer shell occupy then the inner fudge?

Answer 1

0.957 in^3

Explanation:

Answer 2

`0.96\text{ in}^3`

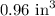
Explanation:

We have been given that Lindor's Truffles have an outer shell diameter of 1.25 in. and a creamy milk chocolate fudge that makes up the inner circle of the ball that has a diameter of 0.5 in.

Since truffles are in shape of sphere, so we will use volume of spherical shells formula to solve our given problem.

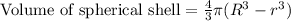`\text{Volume of spherical shell}=(4)/(3)\pi (R^3-r^3)`, where,

`R=\text{Radius of outer shell}`

`r=\text{Radius of inner shell}`

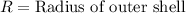
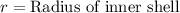
We know that radius of a circle half the diameter of circle. So we need to divide diameters of inner and outer shell by 2 to find their respective radii.

`\text{Radius of outer shell}=(1.25)/(2)=0.625`

`\text{Radius of inner shell}=(0.5)/(2)=0.25`

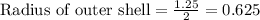
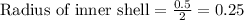
Upon substituting the values of radii in above formula we will get,

`\text{Volume of spherical shell}=(4)/(3)\pi (0.625^3-0.25^3)`

`\text{Volume of spherical shell}=(4)/(3)\pi (0.244140625-0.015625)`

`\text{Volume of spherical shell}=(4)/(3)\pi (0.228515625)`

`\text{Volume of spherical shell}=0.3046875\pi`

`\text{Volume of spherical shell}=0.95720401164\approx 0.96`

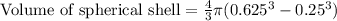
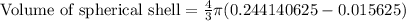
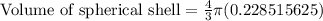
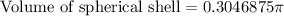
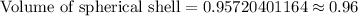
Therefore, the outer shell occupies 0.96 cubic inches of more volume than the inner fudge.