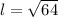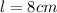ANSWER
A. 8cm
Step-by-step explanation
The diagonal of the square is
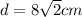
Let the length of each side of the square be l units.
Then the diagonal and any two sides form an isosceles right triangle with the diagonal being the hypotenuse.
We use the Pythagoras Theorem, to obtain,
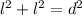
This implies that,


Solve for l,
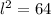
Take positive square root of both sides