Question

Does anyone knows how to solve this?

Answer 1

see explanation

Explanation:

Given a parabola in standard form

y = ax² + bx + c ( a ≠ 0 )

Then the x- coordinate of the vertex is

`x_(vertex)` = -
`(b)/(2a)`

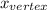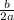
y = x² - 8x + 13 ← is in standard form

with a = 1 and b = - 8 , then

`x_(vertex)` = -
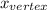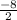`(-8)/(2)` = 4

Substitute x = 4 into the equation for corresponding value of y

y = 4² - 8(4) + 13 = 16 - 32 + 13 = - 3

Vertex = (4, - 3 )

The axis of symmetry is a vertical line passing through the vertex with equation

x = 4 ← equation of axis of symmetry

To determine direction of opening

• If a > 0 then graph opens up

• If a < 0 then graph opens down

Here a = 1, thus graph opens up

Answer 2

This is the answer do it fast. Ok