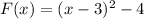Question

the graph of F(x), shown below, has the same shape as the graph of G(x) = x^2, but it is shifted down 4 units and to the right 3 units. What is its equation?

Answer 1

`F(x)=(x-3)^(2)-4`

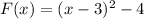
Explanation:

The graph of F(x) is a translation of the graph of G(x) by 4 units down and 3 units right.

`G(x)=x^(2)`

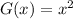
4 units down is the vertical shift. Vertical shift is obtained by adding or subtracting a number from the function value. Subtraction indicates a downward shift and addition indicates an upward shift. Since the graph is shifted 4 units down, the new equation after this translation will be:

`x^(2) - 4`

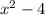
3 units to the right is the horizontal shift. Horizontal shift is obtained by adding or subtracting a number from x. Subtraction indicates a shift towards right and addition indicates a shift towards left. Since the graph is being shifted 3 units to right, the new equation after this transformation will be:

`F(x)=(x-3)^(2)-4`