Answer:
The pair of functions that is not inverse functions are:
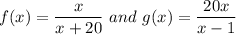
Explanation:
We know that two functions a and b are said to be inverse of each other if:
f(g(x))=g(f(x))=x
i.e. the compositions of the function gives identity.
and the inverse of the given function is calculated by equation the function to y and then calculating the value of x in terms of y.
and the function in terms of y is the inverse function.
A)
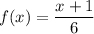
Now if f(x)=y then

B)
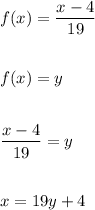
i.e.
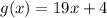
C)
![f(x)=x^5\\\\\\f(x)=y\\\\\\x^5=y\\\\\\x=\sqrt[5]{y}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/8mww2hhr34logy314yhuufi1ozrpnmbd5a.png)
i.e. we have:
![g(x)=\sqrt[5]{x}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/i2sv3m0kgaasknu19pmq47xrvnsw8doukz.png)
D)

if f(x)=y then
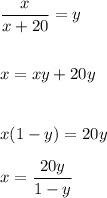
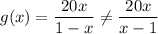
Hence, option: D is the answer.