Question

Consider that the length of rectangle A is 10 cm and its width is 6 cm. Which rectangle is similar to rectangle A? A) A rectangle with a length of 9 cm and a width of 6 cm. B) A rectangle with a length of 15 cm and a width of 9 cm. C) A rectangle with a length of 14 cm and a width of 7 cm. D) A rectangle with a length of 12 cm and a width of 8 cm.

Answer 1

A rectangle with a length of 15 cm and a width of 9 cm.

Explanation:

In similar rectangles, the ratios of the corresponding sides are equal.In similar rectangles, the ratios of the corresponding sides are equal.

`(length)/(width)` →
`(10)/(6)` =
`(5)/(3)` and
`(15)/(9)` =
`(5)/(3)`

Answer 2

B) A rectangle with a length of 15 cm and a width of 9 cm

Explanation:

we know that

If two figures are similar, then the ratio of its corresponding sides is proportional

Verify each cases

case A) A rectangle with a length of 9 cm and a width of 6 cm

`(10)/(9)\\eq (6)/(6)`

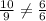
therefore

The rectangle case A) is not similar to rectangle A

case B) A rectangle with a length of 15 cm and a width of 9 cm

`(10)/(15)=(6)/(9)`

`(2)/(3)=(2)/(3)`

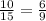
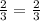
therefore

The rectangle case B) is similar to rectangle A

case C) A rectangle with a length of 14 cm and a width of 7 cm

`(10)/(14)\\eq (6)/(7)`

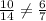
therefore

The rectangle case C) is not similar to rectangle A

case D) A rectangle with a length of 12 cm and a width of 8 cm

`(10)/(12)\\eq (6)/(8)`

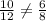
therefore

The rectangle case D) is not similar to rectangle A