Question

Does the graph of the function y–x^2=9 intersect the following. If the answer is ‘yes’, determine the coordinates of the points of intersection the y axis

Answer 1

The function intersects the y-axis at point (0.9) (vertex)

Explanation:

we know that

The equation of a vertical parabola into vertex form is equal to

`y=a(x-h)^(2)+k`

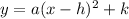
where

(h,k) is the vertex of the parabola

if a>0 then the parabola open upward (vertex is a minimum)

In this problem we have

`y-x^2=9` ----->
`y=x^2+9`

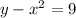This is a vertical parabola open upward

The vertex is the point (0,9)

The vertex is a minimum

see the attached figure to better understand the problem

The graph does not intersect the x-axis (the function has no real roots)

The function intersects the y-axis at point (0.9) (vertex)