Question

Can you help solve this by simplifying the radical?

Answer 1

First of all, we can split the root of the multiplication into the multiplication of the roots:

`\sqrt[3]{-56ab^6c^(10)}=\sqrt[3]{-56}\cdot\sqrt[3]{a}\cdot\sqrt[3]{b^6}\cdot\sqrt[3]{c^10}`

If we look at the prime factorization of -56 we have

`-56=2^3\cdot 7`

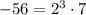
So, we have

`\sqrt[3]{-56} = -\sqrt[3]{2^3\cdot 7} = -\sqrt[3]{2^3}\cdot\sqrt[3]{7} = -2\sqrt[3]{7}`

We can do nothing about
`\sqrt[3]{a}`, because the exponent is lower than the order of the root.

We have

`\sqrt[3]{b^6} = (b^6)^{(1)/(3)} = b^(6)/(3)=b^2`

Finally, we have

`\sqrt[3]{c^(10)} =\sqrt[3]{c^(9+1)}=\sqrt[3]{c^(9)\cdot c}=c^(9)/(3)\cdot\sqrt[3]{c}=c^3\sqrt[3]{c}`

So, the whole expression is equivalent to

`-2b^2c^3\sqrt[3]{7ac}`