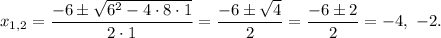Question

The first step in determining the solution to the system of equations, y = –x^2 – 4x – 3 and y = 2x + 5, algebraically is to set the two equations equal as –x^2 – 4x – 3 = 2x + 5. What is the next step?

Answer 1

The next step is to put all terms into the left side of the equation and group the like trems

Explanation:

The first step in determining the solution to the system of equations,
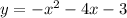`y =-x^2-4x-3` and
`y = 2x + 5`, algebraically is to set the two equations equal as
`-x^2-4x-3 = 2x + 5.`

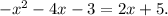
The next step is to put all terms into the left side of the equation and group the like trems:

`-x^2-4x-3-2x-5=0,\\ \\-x^2+(-4x-2x)+(-3-5)=0,\\ \\-x^2-6x-8=0.`

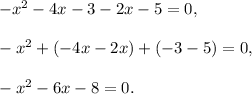
Now you can multiply this equation by -1:

`x^2+6x+8=0`

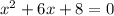
and solve it using quadratic formula:

`x_(1,2)=(-6\pm √(6^2-4\cdot 8\cdot 1))/(2\cdot 1)=(-6\pm√(4))/(2)=(-6\pm 2)/(2)=-4,\ -2.`