Question

A can of tuna fish is a cylinder with a height of 1.75 inches and a diameter of 3 inches. If the can is half full, how many cubic inches of tuna is in the can?

Answer 1

Explanation:

jaz its alexis

Answer 2

Answer:
`6.185in^3`

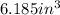
Explanation:

The formula for calculate the volume of a cylinder is:

`V_(cylinder)=\pi r^2h`

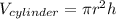
r is the radius of the cylinder and h is the height of the cylinder.

Given the diameter of 3 inches, calculate the radius of the cylinder with:

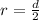`r=(d)/(2)` (Where d is the diameter)

`r=(3in)/(2)=1.5in`

Knowing the height and the radius, you can calculate the volume of the entire can of tuna:

`V_(can)=\pi (1.5)^2(1.75in)=12.37in^3`

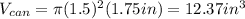
As you want to know the cubic inches of tuna that are in the can (volume of tuna), and you know that the can is half full, then:

`V_(tuna)=(V_(can))/(2)\\\\V_(tuna)=(12.37in^3)/(2)\\\\V_(tuna)=6.185in^3`