Question

Need help with this question

Answer 1

Option 1: 28 is the correct answer.

Explanation:

The given expression for sum is:

`\[ \sum_(n=1)^(4) {2n+2} = 1 \]`

There will be four terms in the sum

We have to find the terms by putting n=1,2,3,4 as n=1 to 4

Putting n=1

`=2(1)+2 = 2+2 = 4`

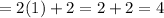
Putting n=2

`=2(2)+2 = 4+2 = 6`

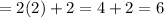
Putting n=3

`=2(3)+2 = 6+2 = 8`

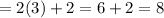
Putting n=4

`=2(4)+2 = 8+2 = 10`

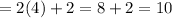
So the summation will expand to:

`\[ \sum_(n=1)^(4) {2n+2} = 1 \] = 4+6+8+10 = 28`

Hence,

Option 1: 28 is the correct answer.