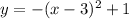Question

What is the equation of the graph below?
​

Answer 1

The equation of the graph is y= -(x+3)^2 +1

Choice A is the correct answer

Answer 2

The equation of the given graph is:

`y=-(x-3)^2+1`

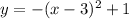
Explanation:

We know that the general equation of a downward parabola with vertex at (h,k) is given by the formula:

`y=a(x-h)^2+k`

where a<0

Here in each of the options we have a= 1 or -1

but as a has to be less than 1 this means that a= -1

Also, we have: (h,k)= (3,1)

i.e. h=3 and k=1

Hence, putting the values of h,k and a in the general equation we get:

Hence, the equation of parabola is:

`y=-(x-3)^2+1`