Answer:

Explanation:
The problem tells us that the slope of the tangent to a curve at any point
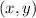 on the curve is x divided by y, that is:
on the curve is x divided by y, that is:
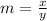
We also know that the point
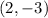 is on the curve. By taking a look on the options this point lies on both equations, namely:
is on the curve. By taking a look on the options this point lies on both equations, namely:

We know that the derivative is the slope of the tangent line to the graph of the function at a given point. So taking the derivative of both equations we have:

And:
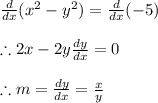
So
 also meets the requirement of the condition the slope of the tangent to a curve at any point (x, y) on the curve is x divided by y. Therefore this is the correct option.
also meets the requirement of the condition the slope of the tangent to a curve at any point (x, y) on the curve is x divided by y. Therefore this is the correct option.