Answer:
The height of the hurdler when he clears the hurdle is approximately 1.1045 meters (high)
Step-by-step explanation:
The given parameters are;
The distance the hurdler is from the hurdle, d = 0.535 m
The velocity with which the hurdler jumps, u = 6.82 m/s
The angle at which the hurdler jumps, θ = 67.9°
The horizontal component of the velocity is uₓ = u × cos(θ)
∴ uₓ = 6.82 × cos(67.9°) ≈ 2.566 m/s
The time it takes, t, the hurdler to get to the hurdle horizontally 0.535 m away is given as follows;
Time, t = Distance/Velocity = d/uₓ = 0.535 m/(2.566 m/s) ≈ 0.2085 s
t ≈ 0.2085 s
The kinematic equation of vertical motion, under gravity can be presented as follows;
h =
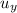·t - 1/2·g·t²
Where;
h = The height reached by the hurdler in time t
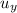= The vertical component of the velocity = u × sin(θ)
g = The acceleration due to gravity
∴
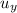= 6.82 m/s × sin(67.9°) ≈ 6.3189 m/s
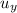≈ 6.3189 m/s
Substituting the known values gives;
h = 6.3189 × 0.2085 - 1/2×9.8×0.2085² ≈ 1.1045 m
The height of the hurdler when he clears the hurdle, h ≈ 1.1045 m.