Question

Force F acts between a pair of charges, q1 and q2, separated by a distance d. For each of the statements, use the drop-down menus to express the new force in terms of F.

q1 is halved, q2 is doubled, but the distance between the charges remains d.
F/4
F
6F
6F/4


q1 and q2 are unchanged. The distance between the charges is doubled to 2d.
F/4
F
6F
6F/4

q1 is doubled and q2 is tripled. The distance between the charges remains d.
F/4
F
6F
6F/4

Answer 1

q1 is halved, q2 is doubled, but the distance between the charges remains d.

✔ F
q1 and q2 are unchanged. The distance between the charges is doubled to 2d.

✔ F/4

q1 is doubled and q2 is tripled. The distance between the charges remains d.

✔ 6F

Step-by-step explanation:

Answer 2

The initial force between the two charges is given by:

`F=k (q_1 q_2)/(d^2)`

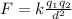
where k is the Coulomb's constant, q1 and q2 the two charges, d their separation. Let's analyze now the other situations:

1. F

In this case, q1 is halved, q2 is doubled, but the distance between the charges remains d.

So, we have:

`q_1' = (q_1)/(2)\\q_2' = 2 q_2\\d' = d`

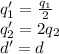
So, the new force is:

`F'=k (q_1' q_2')/(d'^2)= k (((q_1)/(2))(2q_2))/(d^2)=k (q_1 q_2)/(d^2)=F`

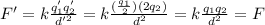
So the force has not changed.

2. F/4

In this case, q1 and q2 are unchanged. The distance between the charges is doubled to 2d.

So, we have:

`q_1' = q_1\\q_2' = q_2\\d' = 2d`

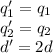
So, the new force is:

`F'=k (q_1' q_2')/(d'^2)= k (q_1 q_2))/((2d)^2)=(1)/(4) k (q_1 q_2)/(d^2)=(F)/(4)`

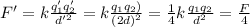
So the force has decreased by a factor 4.

3. 6F

In this case, q1 is doubled and q2 is tripled. The distance between the charges remains d.

So, we have:

`q_1' = 2 q_1\\q_2' = 3 q_2\\d' = d`

So, the new force is:

`F'=k (q_1' q_2')/(d'^2)= k ((2 q_1)(3 q_2))/(d^2)=6 k (q_1 q_2)/(d^2)=6F`

So the force has increased by a factor 6.