Answer:
(x-1)²+(y-1)² = 37 is the equation of circle.
Explanation:
We have given the center (1,1) and a point P (2,-5) from which the circle is passes.
So, the radius of the circle is :
r=
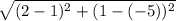
r =
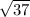
The equation of circle is :
(x - x₁)² +(y - y₁)² = r² where (x₁,y₁) is the center of circle.
Putting the value in above equation we get,
(x-1)²+(y-1)² = (√37)²
(x-1)²+(y-1)² = 37 is the equation of circle.