Answer:
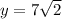
Explanation:
Notice that the problem is about a right triangle, where the opposite leg is 7 and the acute angle is 45°.
This is an special right triangle because it has acute angles of 45°, that means the legs are congruent, because it's a symmetric right triangle. Basically, when a right triangle has acute angles of 45°, it means is an isosceles triangle too.
Additionally, a special right triangle like this has a pattern, its hypothenuse is
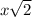 , where
, where
 is the length of a leg.
is the length of a leg.
So, in this case, the hypothenuse is
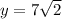
And the missing leg is
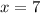 .
.