Question

Solve √5r - 9 - 3 = √r +4-2

Answer 1

r = 5

Explanation:

Solving radical equations often results in extraneous solutions. These can be avoided by using a graphing calculator for the solution.

Graph

The attached shows that the value of r that makes both sides of the equation have the same value is r = 5.

Check:

√(5·5 -9) -3 = √(5 +4) -2 ⇒ 4 -3 = 3 -2 . . . true

Analytical solution

Solution of an equation like this is typically done by isolating the radical expressions, then squaring the equation.

We can add 3, square the equation, then isolate the radical and square again:

`\displaysyle √(5r-9)-3=√(r+4)-2\\\\√(5r-9)=√(r+4)+1\qquad\text{add 3}\\\\5r-9=(r+4)+2√(r+4)+1\qquad\text{square both sides}\\\\(4r-14)^2=4(r+4)\qquad\text{subtract $(r+5)$ and square again}\\\\16r^2-116r+180=0\qquad\text{rewrite in standard form}\\\\4(4r -9)(r -5) = 0\qquad\text{factor}\\\\r=\{(9)/(4),\ 5\}\qquad\text{solutions to the factored form}`

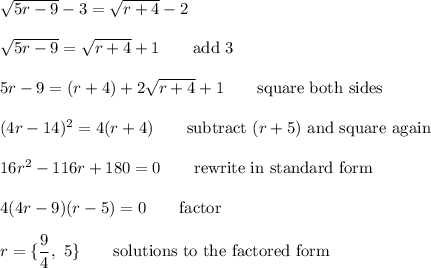
Trying these values in the original equation, we get ...

√((5(9/4) -9) -3 = √(9/4 +4) -2 ⇒ 1.5 -3 = 2.5 -2 . . . . . false

√(5(5) -9) -3 = √(5 +4) -2 ⇒ 4 -3 = 3 -2 . . . . . true

The solution is r = 5.