Question

What is the sum of series is numbers 64&66 ?
Using the summation notation

Answer 1

64. 6138

66. 8.078125

Explanation:

Let's take it one number at a time.

For number 64:

`6(2)^(1-1)=6`

`6(2)^(2-1)=12`

`6(2)^(3-1)=24`

`6(2)^(4-1)=48`

`6(2)^(5-1)=96`

`6(2)^(6-1)=192`

`6(2)^(7-1)=384`

`6(2)^(8-1)=768`

`6(2)^(9-1)=1536`

`6(2)^(10-1)=3072`

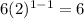
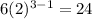
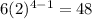
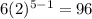
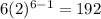
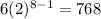
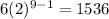
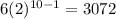
Sum = 6 + 12 + 24 + 48 + 96 + 192 + 384 + 768 + 1536 + 3072

Sum = 6138

For number 66:

`12(-(1)/(2))^(0)=12`

`12(-(1)/(2))^(1)=-6`

`12(-(1)/(2))^(2)=3`

`12(-(1)/(2))^(3)=-1.5`

`12(-(1)/(2))^(4)=0.75`

`12(-(1)/(2))^(5)=-0.375`

`12(-(1)/(2))^(6)=0.1875`

`12(-(1)/(2))^(7)=-0.0078125`

`12(-(1)/(2))^(8)=0.046875`

`12(-(1)/(2))^(9)=-0.0234375`

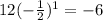
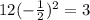
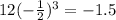
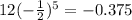
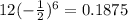
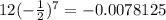
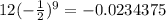
Sum = 12 + -6 + 3 + -1.5 + 0.75 + -0.375 + 0.1875 + -0.0078125 + 0.046875 + -0.0234375

or to make things simpler:

Sum = 12 - 6 + 3 - 1.5 + 0.75 - 0.375 + 0.1875 - 0.0078125 + 0.046875 - 0.0234375

Sum = 8.078125