Question

`f(x) = (x^(2) +4x-4)/(x^(2) -2x-8)`

Domain:
V.A:
Roots:
Y-int:
H.A:
Holes:
O.A:

Also, draw on the graph attached.

Answer 1

i) The given function is

`f(x)=(x^2+4x-4)/(x^2-2x-8)`

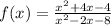
We can rewrite in factored form to obtain;

`f(x)=(x^2+4x-4)/(x^2-2x-8)`

`f(x)=((x+2√(2)+2)(x-2√(2)+2))/((x-4)(x+2))`

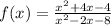
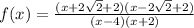
The domain is

`(x-4)(x+2)\\e0`

`(x-4)\\e0,(x+2)\\e0`

`x\\e4,x\\e-2`

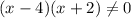
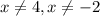
ii) To find the vertical asymptotes equate the denominator to zero.

`(x-4)(x+2)=0`

`(x-4)=0,(x+2)=0`

`x=4,x=-2`

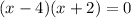
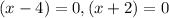
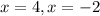
iii) To find the roots, equate the numerator to zero.

`(x+2√(2)+2)(x-2√(2)+2)=0}`

`(x+2√(2)+2)=0,(x-2√(2)+2)=0}`

`(x=-2√(2)-2,x=2√(2)-2)}`

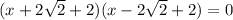
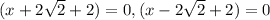
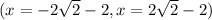
iv) To find the y-intercept, substitute
`x=0` into the equation.

`f(0)=(0^2+4(0)-4)/(0^2-2(0)-8)`

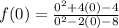
We simplify to obtain;

`f(0)=(-4)/(-8)`

`f(0)=(1)/(2)`

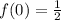
v) The horizontal asymptote is

`lim_(\to \infty)(x^2+4x-4)/(x^2-2x-8)=1`

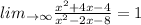
The equation of the horizontal asymptote is y=1

vi) The function does not have a variable factor that is common to both the numerator and the denominator.

The function has no holes in it.

vii) The given function is a proper rational function.

Proper rational functions do not have oblique asymptotes.